World Logic Day

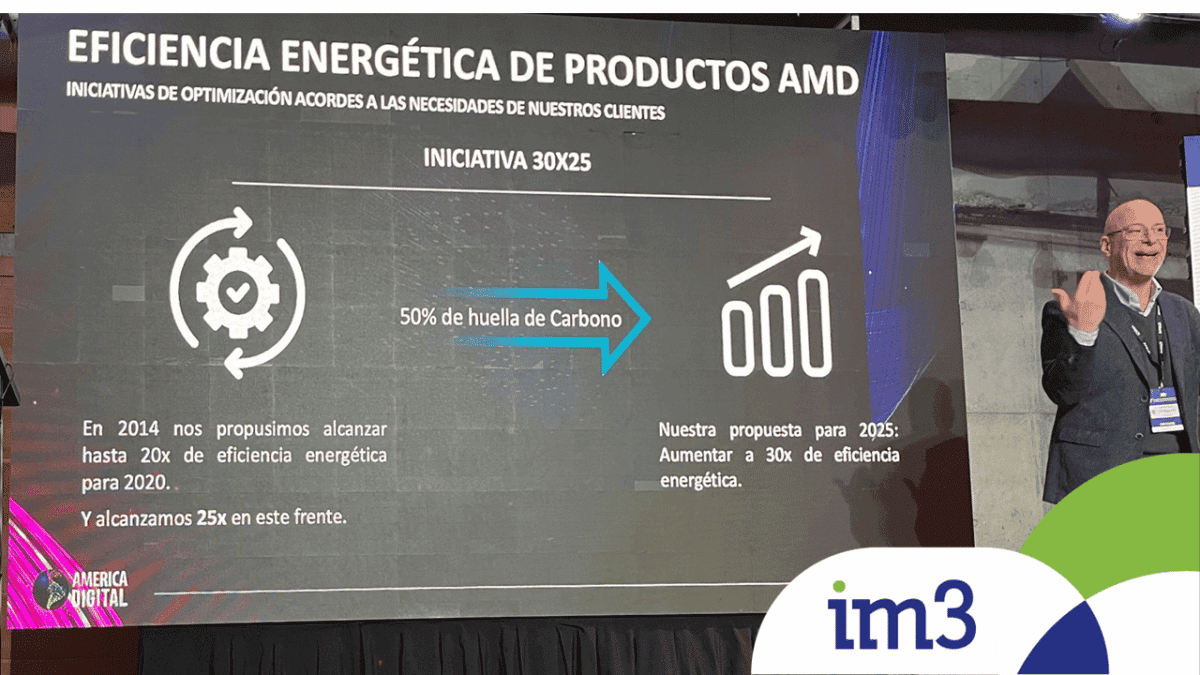
At Im3 we continue to celebrate the World Logic Day for the second consecutive year because logic is simply great.

And we continue to use the Pythagorean Theorem as an example because of how paradoxically simple and complex it is at the same time.

Millions of people simply by looking long enough at the symbols and squares in the image above, without the need for further explanation, could come to understand the world’s best-known Theorem, whose equation a2=b2+c2 rivals in fame with Einstein’s E= mc2.

And both equations share another characteristic: a great simplicity that hides some very deep fundamental laws.

If we draw a circle with centre at the intersection b-c and radius equal to c, we can begin to develop all the ratios and trigonometric properties by deduction and logical development, until we define the sinusoidal forms with which Wave Functions can be represented, which are the most basic way to describe any object in Quantum Mechanics.

Sinusoidal functions can also represent the propagation of fields, including the electromagnetic one, which if it has the appropriate wavelength will be visible in the form of light, whose speed is equal to c, the universal constant of Einstein’s equation that formulates the equivalence between energy E and mass m.

Returning to the Pythagorean Theorem, which we have formulated for two dimensions, it is also valid in its three-dimensional form, for example, for the surface of a sphere like the Earth, according to the following equation: R2=x2+y2+z2. In his General Theory of Relativity, Einstein added the time dimension T, validating that the equation x2+y2+z2-T2 is a four-dimensional space-time version of the Pythagorean Theorem.

What we have tried to show in this post is that we have logic at our fingertips for all levels of difficulty, from zero to infinity and beyond:

“The world is simple and complex, logical and strange, orderly and chaotic” Frank Wilczek